Semiclassical transport through open quantum billiards
(with L. Wirtz, I. Brezinova, S. Rotter, and J. Burgdörfer)
Semiclassical mechanics represents one of the most powerful tools to gain an
intuitive understanding of transport effects in mesoscopic systems.
Moreover, semiclassical techniques allow to bridge classical and quantum
mechanics in a very direct way: the classical paths are carrying an amplitude
which reflects the geometric stability of the orbits and
a phase that contains the classical action and accounts for
effects of quantum interference.
Ballistic transport through billiards has been studied extensively in the last
decade and a variety of semiclassical approximations
has been introduced in order to provide a qualitative and partly also a
quantitative understanding of these systems.
In particular, universal conductance fluctuations (UCF) and
"weak localization" (WL) have been studied in order to delineate
characteristic differences in the quantum transport of classically
chaotic and integrable billiards.
While in full quantum mechanics, propagation between the leads of
the billiard proceeds via all possible paths,
semiclassical theory reduces the propagator
to a sum of classical paths connecting the two leads.
However, related to this restriction,
semiclassical approximation have revealed systematic problems in
approximating quantum transport. In particular, the semiclassical
S-Matrix displays a violation of unitarity which - in some cases -
exceeds the typical conductance fluctuations that are to be described.
We quantitatively investigate the performance of semiclassical
approximations for transport in mesoscopic systems and
show how a systemic inclusion of diffraction effects leads to convergence
towards quantum transport.
Text from L. Wirtz.
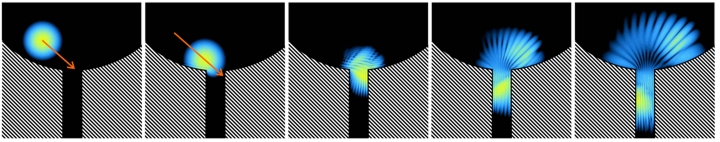FIGURE - An electron wavepaket scatterd at an open lead in a circular quantum billiard. By courtesy of Iva Brezinova and Johannes Feist.
[1] Semiclassical theory for transmission through
open billiards: Convergence towards quantum transport;
L. Wirtz, C. Stampfer, S. Rotter, and J. Burgdörfer,
Phys.
Rev. E
67 016206 (2003)
[2] Pseudo-path semiclassical approximation to transport through open quantum billiards:
Dyson equation for diffractive scattering;
C. Stampfer, L. Wirtz, S. Rotter, and J. Burgdörfer, Phys.
Rev. E
72 036223 (2005).
[3] Diffractive paths for weak localization
in quantum billiards;
I. Brezinova, C. Stampfer, L. Wirtz, S. Rotter, and J. Burgdörfer, arXiv:0709.3210v1, (2007).
|
